Scribe Post, Identities
Good evening fellow students, names Steve =o. I hope you guys enjoyed the formulas and the 'plug and solve' methods on previous units because it will be rarely used in this unit, Identities. Like Mr.K mentioned, practice is what will be the key to you mastering these problems, so the beginning of the first class of todays two-class day he threw at us these problems:
Examples & Solutions:
Now, that wasn't so bad. Unfortunetly, as always they get tougher >_>;

Fear my fish/Alpha variables =P
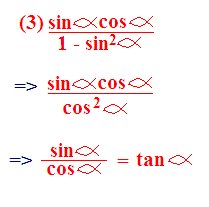

Fear my fish/Alpha variables =P
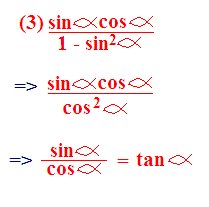

Well, goody. Those were the simplification problems, now that they're done with, we have some questions where we have to prove that one operation equals the other. Im kind of running behind schedual, those pictures were hard to put together =P, thats was fun, but lets see if we can deal with simple text typed ones.
Examples: Prove!
1)secx - cosx = sinx+tanx
If starting with the Left side:
=>(1/cosx)-cosx
=>(1/cosx)-(cos2x/cosx)
=>(1/cosx)-(cos2x/cosx)
=>(1-cos2x/cosx)
=>(sin2x/cosx)
=>sinx(sinx/cosx)
=>sinxtanx <,/> <= a check mark =P, squint! You might see it.
If starting with the Right side:
=>sinx(sinx/cosx)
=>(sin2x/cosx)
=>(1-cos2x/cosx)
=>(1/cosx) - (cos2x/cosx)
=>(1/cosx) - cosx
=>secx - cosx <,/>
2)[1/(1-sinx)] - [1/(1+sinx)] = 2tanxsecx
Apparently, we learned an operation would equal another operation if their result is the same, and it DOES NOT have to look like one another, like this example here.
If starting with the Left side:
=>[(1+sinx) - (1-sinx)] / [(1-sinx)(1+sinx)]
=>2sinx/(1-sin2x)
=>2sinx/(1-sin2x)
=>2sinx/cos2x <,/>
If starting with the Right side:
=>2(sinx/cosx)(1/cosx)
=>2sinx/cos2x <,/>
Well, that was the end of the first class. It was hard to get used to, so usually alot of explaination was needed to help understand Identities more, which is why we only had 6 questions. Great! you got this far, it's intermission time =P. It was lunch for us, go get a snack or something and come back.

Welcome back! Being seated we noticed Mr.K had put up more questions for our... pleasure? Well, lets get to it! Noted, he has raised the bar once more, these were much tougher for us, noticably.
Examples: Prove!
(secx-1)/sin2x = sec2x/(1+secx)
For example: (1/cosx) + 1, representing 1 as (cosx/cosx) is completely legit! This technique can be crucial to figuring out the Identity! As shown in this example.
If starting with the Left side:
=>[(1/cosx)-1]/(1-cos2x)
=>[(1/cosx)-(cosx/cosx)]/(1-cos2x)
=>[(1-cosx)/cosx]/(1-cos2x)
=>[(1-cosx)/cosx] x [1/(1-cos2x)]
=>(1-cosx)/[cosx(1+cosx)(1-cosx)]
=>1/[cosx(1+cosx)] <,/>
If starting with the Right side:
=>(1/cos2x)/[1+(1/cosx)]
=>(1/cos2x)/[(cosx/cosx)+(1/cosx)]
=>(1/cos2x)/[(cosx+1)/cosx)
=>(1/cos2x)/[cosx/(cos2x+1)]
3)[1/(1+sinx)] + [1/(1-sinx)] = 2sec2x
This question is a good example which side is best to pick. Because sometimes a side maybe really hard to figure out, you'll see why in a moment.
If starting with the Left side:
=>[(1-sinx) - (1+sinx)]/[(1+sinx)(1-sinx)]
=>[1-sinx+1+sinx]/(1-sin2x)
=>2(1/cos2x)
=>2sec2x
If starting with the Right side:
=>2(1/cos2x)
=>2/cos2x
Now, this is the part where if people were to pick the right side will be stuck on. Givin the evidence on the left side, 2/cos2x would = [1-sin0+1+sin0]/(1-sin20). Yes that it is true it works out, but would anyone really realize 2/cos2x would = [1-sin0+1+sin0]/(1-sin20) if it havent been for them finding the left hand side first? Meh, *probably* not, so this is a good example why picking the most complicated side first, because you can break it down more easily than building it back up. It makes sense here and in real life too! Is it easier to hop down a cliff (support of course =p) than to climb it?
The last part of the class was inputing stuff into our math Dictionary, enjoy!
Trigonometric Identities
The Fundemental Identities
Tanx = sinx/cosx
Cotx = cosx/sinx
Secx = 1/cosx
Cscx = 1/sinx
The Pythagorean Identities
[sin2x+cos2x=1]]
Corollaries
sin2x=1-cos2x
cos2x=1-sin2x
(sin2x/sin2x) + (cos2x/cos2x) = (1/sin2x)
[1+cot2x = csc2x]
Corollaries
cot2x=csc2x-1
1=csc2x-cot2
(sin2x/cos2x) + (cos2x/cos2x) = (1/cos2x)
[tan2x+1=sec2x]
Corollaries
1=sec2x-tan2x
tan2x=sec2x-1
Even and Odd Identities
sin(-x) = -sinx
cos(-x) = -cosx
tan(-x) = -tanx
Strategies for Solving Trig Identities
(1)Work with the 'more complicated' side first
(2)Rewrite both sides of the equation in terms of sine and cosine
(3)Use Pythagoreal Identities to make appropriate substitution
(4)COmbine Functions
(5)Use Factoring (especially difference of squares)


Hi there!
ReplyDeleteYour graphs and notes are so very helpful. Your writing made me feel as I was right there talking to you. And your intermission and graphical illustration of "more than one way" a perfect addition!
I wonder if this post isn't worthy of "The Hall of Fame"?
Best,
Lani
This is an awesome post. I really enjoyed,
ReplyDeleteNow, this is the part where if people were to pick the right side will be stuck on.
This type of advise is what separates regular scribe posts from hall of fame ones. Come on Pre-Cal 40s. Have your say. Did ScubaSteve scribe his way in. You have to vote through your comments.
Mr. Harbeck
Sargent Park School
i enjoyed reading the post especially the "intermission" and the "cat thingy"! I think it should be in the hall of fame!
ReplyDeleteWelocome to the Hall of Fame!!
ReplyDeletethis post is no doubt, belongs to the hall of fame! neatly done and organize. bienvenue au hall de la renommée!! (welcome to the hall of fame!)
ReplyDelete